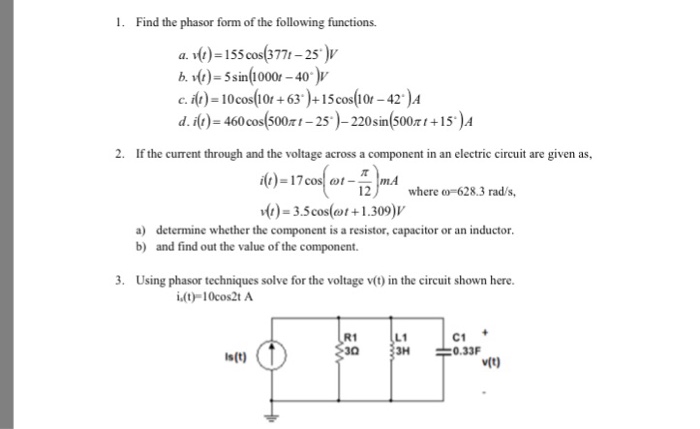
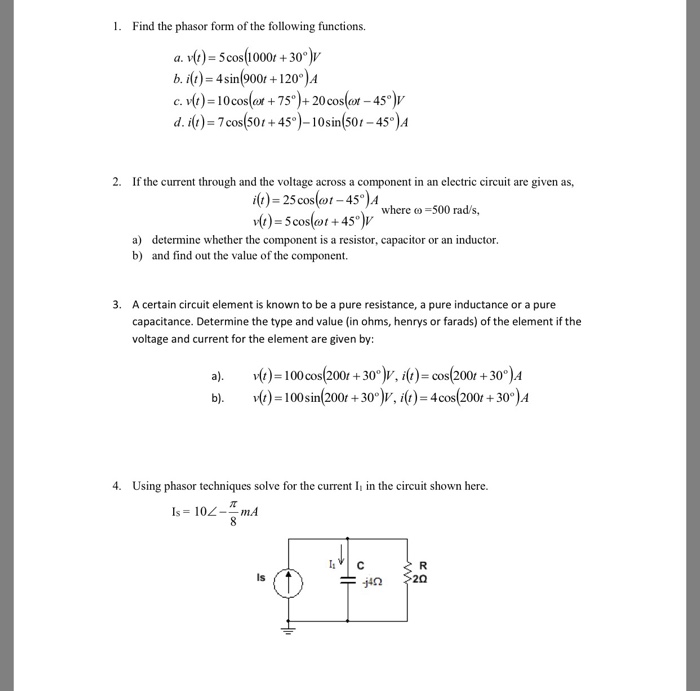
Find the phasor form of the following functions – Delving into the realm of electrical engineering, this discourse embarks on a journey to explore the concept of phasor representation and its significance in analyzing sinusoidal functions. By harnessing the power of complex numbers, we unravel the intricacies of phasor diagrams, unlocking their potential in circuit analysis and beyond.
Phasor representation provides a powerful tool for representing sinusoidal functions, enabling engineers to simplify complex calculations and gain insights into the behavior of electrical circuits. This comprehensive guide delves into the theoretical foundations and practical applications of phasor analysis, equipping readers with a deep understanding of this essential technique.
Phasor Representation

Phasor representation is a graphical technique used to represent sinusoidal functions in a complex plane. It simplifies the analysis of AC circuits by converting sinusoidal functions into vectors with magnitude and phase angle. This representation provides a convenient way to visualize and manipulate AC quantities.
Examples of Phasor Representation in Electrical Engineering
- Representing voltage and current in AC circuits
- Analyzing power factor and impedance
- Solving circuit problems involving AC sources
Sinusoidal Functions: Find The Phasor Form Of The Following Functions
Sinusoidal functions are periodic functions that describe the variation of a quantity over time. They are characterized by their amplitude, frequency, and phase angle.
Phasor Form of Sinusoidal Functions
The phasor form of a sinusoidal function is a complex number that represents the magnitude and phase angle of the function. It is given by:
V = Vm∠θ
where:
- V is the phasor representation
- V mis the amplitude
- θ is the phase angle
Complex Numbers
Complex numbers are numbers that have both a real and an imaginary part. They are represented as:
z = a + bi
where:
- a is the real part
- b is the imaginary part
- i is the imaginary unit (i 2= -1)
Conversion Between Sinusoidal Functions and Complex Numbers
The phasor form of a sinusoidal function can be converted to a complex number using Euler’s formula:
V = Vmcos(ωt + θ) = V m(cosθ + isinθ)
Phasor Diagrams

Phasor diagrams are graphical representations of phasors. They are used to visualize the relationships between different phasors in a circuit.
Construction of Phasor Diagrams
Phasor diagrams are constructed by plotting the phasors on a complex plane. The magnitude of each phasor is represented by the length of the vector, and the phase angle is represented by the angle between the vector and the horizontal axis.
Use of Phasor Diagrams in Circuit Analysis
Phasor diagrams are used to analyze AC circuits by simplifying the calculations. They can be used to determine the voltage, current, power, and impedance in a circuit.
Applications of Phasor Analysis
Phasor analysis is widely used in electrical engineering for the analysis and design of AC circuits.
Applications in Power Systems, Find the phasor form of the following functions
- Power flow analysis
- Voltage regulation
- Stability analysis
Applications in Electronics
- Filter design
- Amplifier analysis
- Oscillator design
FAQ Insights
What is the significance of phasor representation?
Phasor representation simplifies the analysis of sinusoidal functions, enabling engineers to represent both magnitude and phase angle in a single complex number.
How are sinusoidal functions represented in phasor form?
Sinusoidal functions are represented in phasor form using complex numbers, where the magnitude corresponds to the peak amplitude and the angle corresponds to the phase shift.
What is the role of complex numbers in phasor analysis?
Complex numbers provide a convenient mathematical framework for representing and manipulating phasors, allowing for the simplification of complex calculations.