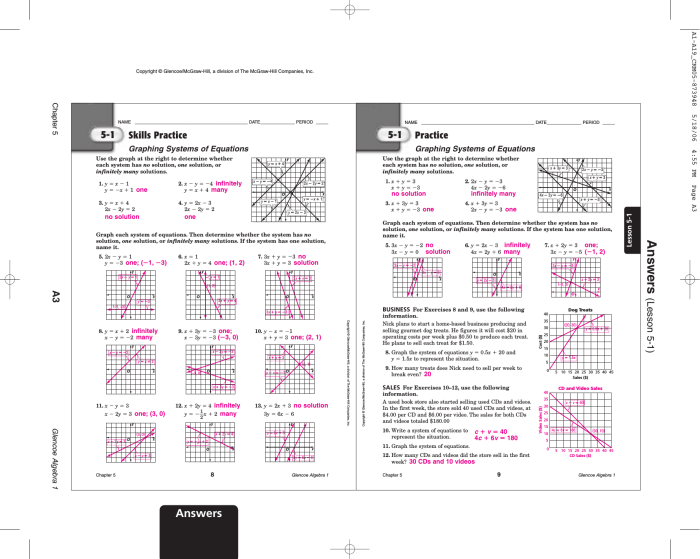
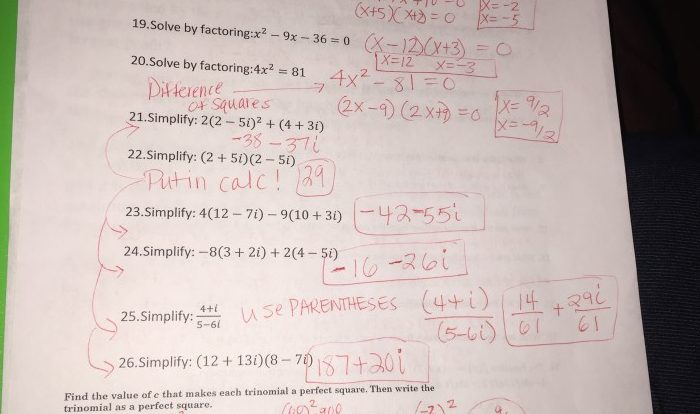Unit 2 test study guide equations and inequalities answer key – Mastering equations and inequalities is a crucial step in mathematical development. This study guide provides a comprehensive overview of these concepts, offering clear explanations, practical strategies, and a detailed answer key to guide you towards success in Unit 2.
Within this guide, you’ll find an in-depth review of equations and inequalities, covering their definitions, types, and solution techniques. Additionally, a comprehensive list of topics covered in Unit 2 is provided, along with key concepts, formulas, and practice problems to enhance your understanding.
Equations and Inequalities Review: Unit 2 Test Study Guide Equations And Inequalities Answer Key
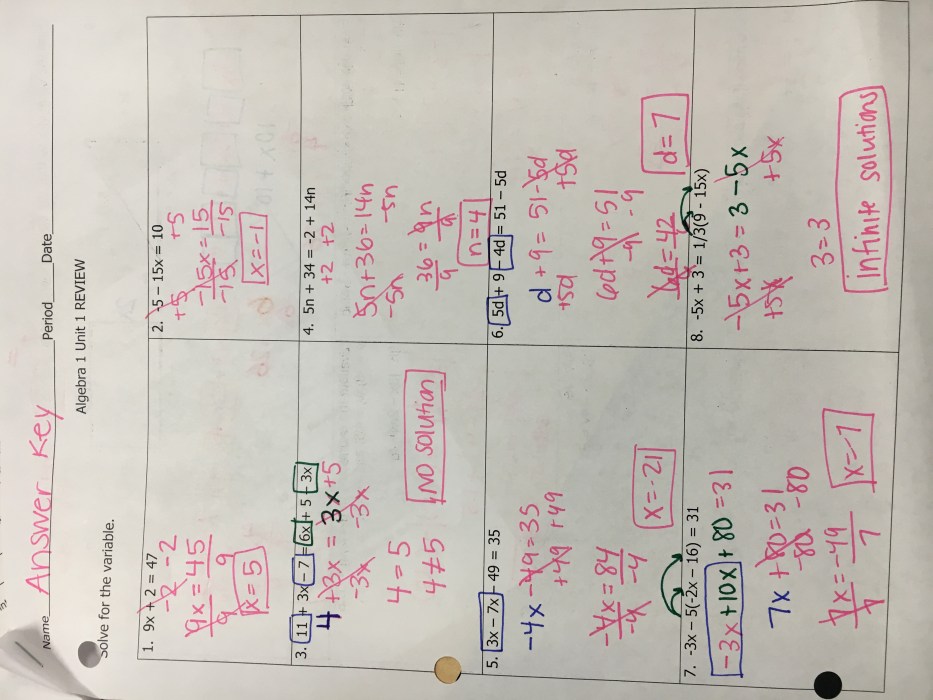
Equations and inequalities are essential mathematical concepts that are used in a wide variety of applications. In this review, we will explain the concept of an equation and provide examples, define inequality and explain the different types, and share strategies for solving equations and inequalities.
Equations
An equation is a mathematical statement that two expressions are equal. Equations can be used to represent a wide variety of relationships, such as the relationship between the length and width of a rectangle or the relationship between the speed of an object and the time it takes to travel a certain distance.
Here are some examples of equations:
- x + 5 = 10
- y – 3 = 7
- 2x + 3y = 12
Inequalities, Unit 2 test study guide equations and inequalities answer key
An inequality is a mathematical statement that two expressions are not equal. Inequalities can be used to represent a wide variety of relationships, such as the relationship between the ages of two people or the relationship between the temperatures of two different cities.
There are three types of inequalities:
- Less than (<): a < b
- Greater than (>): a > b
- Less than or equal to (≤): a ≤ b
- Greater than or equal to (≥): a ≥ b
Solving Equations and Inequalities
There are a variety of strategies that can be used to solve equations and inequalities. Some of the most common strategies include:
- Solving equations by isolating the variable:This strategy involves isolating the variable on one side of the equation by adding, subtracting, multiplying, or dividing both sides of the equation by the same number.
- Solving inequalities by isolating the variable:This strategy involves isolating the variable on one side of the inequality by adding, subtracting, multiplying, or dividing both sides of the inequality by the same number.
- Solving equations and inequalities using graphs:This strategy involves graphing both sides of the equation or inequality and finding the point where the graphs intersect. The point of intersection is the solution to the equation or inequality.
Questions and Answers
What types of equations are covered in this guide?
This guide covers various types of equations, including linear equations, quadratic equations, and systems of equations.
How can I use this study guide effectively?
To effectively use this guide, begin by reviewing the concepts and strategies presented. Practice solving the problems provided, and refer to the answer key for guidance. Additionally, identify areas where you need further clarification and seek support from your instructor or a tutor.